Details of large-panel buildings seismic analysis

1. Introduction

During the design of structures for construction in seismic regions it is necessary to follow the basic requirements developed to reduce the risk of collapses during the earthquakes and to insure the earthquake resistance of buildings. These requirements are based on years of experience analyzing the consequences of catastrophic earthquakes and improvement of anti-seismic measures given in design norms of different countries (Construction in seismic regions of Ukraine, 2006; Seismic Building Design Code, 2011; Structural Engineering Design Provisions, 1997; Eurocode 8, 2004).

Depending on the degree of structures and facilities destruction, some basic principles are developed to insure safety of buildings and facilities designed and constructed in seismic regions (Nemchynov, Yu, 2008; Nemchynov, Yu, Khavkin, Maryenkov, Babik, 2012). They are based on the following principles (Seismic Evaluation and Retrofit of Concrete Buildings, 1996; NEHRP, 1997b; ASCE, 2000):

1. At rare destructive earthquakes it is necessary to insure the safety of people`s lives, valuable equipment and infrastructure which is necessary to eliminate the consequences of earthquakes. The facility can have a limit state close to collapse. This principle is called as Principle of facility safety.

2. At strong earthquakes and earthquakes of moderate intensity the structures can have significant damages and residual deformations. The load-bearing structures should have capability to be stable during the further earthquake (aftershock) without violation of stability. It is the Principle of allowed damages.

3. At weak repetitive earthquakes and limited destructions the approved anti-seismic measures should insure the normal facility operation. It is the Principle of no damages.

At design for earthquake resistance in addition to basic principles it is necessary to do the following actions:

to consider the secondary factors such as fire, displacements or soil liquefaction and others

to assess the response spectra in places where the equipment which is important for facility operation is installed

to develop the measures on population safety including the fire protection, air-conditioning, water supply and other systems

to develop the measures on facility protection against progressive collapse caused by failure of responsible structures, terrorist intervention and other dangerous events

2. Metodology

Main principles to design the structures with expected level of earthquake resistance

Modern methods of earthquake-resistant buildings are based on new approaches which are given in normative documents of the following foreign countries: the USA, Canada, Japan and Europe. The approved approach for design which is called “Performance based seismic engineering”, can be considered as “Design of earthquake-resistant structures with the given parameters of earthquake resistance” or “Design based on performance characteristics”. The most widespread calculation method in this approach is “Nonlinear pushover analysis”. The recommendations for design based on performance characteristics are given in Manuals of Applied Technologies Council of the USA (ATC-40) (Seismic Evaluation and Retrofit of Concrete Buildings, 1996), Federal Emergency Management Agency (FEMA) (NEHRP, 1997a,1997b; ASCE, 2000) and Structural Engineers Association of California (SEAOC) (A framework for performance-based design, 1995).

Figure 1 shows the load-bearing capacity curve graph which represents a new approach to assess the performance characteristics of the existing buildings and to design the buildings with the expected level of earthquake resistance.

Fig. 1:

Relations between summarized forces and displacements for different operation levels corresponding to plastic facility load-bearing capacity curve.

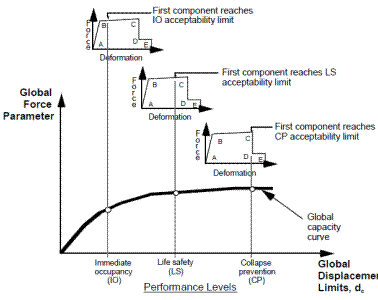
In this case the load-bearing capacity curve is intrinsic (skeleton) for hysteresis curves at cyclic load. In many publications and Instructions on design (NEHRP, 1997b; Prestandard and commentary for the seismic rehabilitation of buildings, 2000; A framework for performance-based design, 1995) there are three variants to idealize the skeleton curve characterizing the dependence between summarized forces F and summarized displacements D.

The variants of the curves correspond to plastic, partially-plastic and fragile behavior of structures destruction. The points A, B, C, D, E on the curves show the levels of plastic state and deformations values. In Manuals (Seismic Evaluation and Retrofit of Concrete Buildings, 1996; Prestandard and commentary for the seismic rehabilitation of buildings, 2000) there are recommendations on selection of skeleton curves parameters corresponding to work of metal, reinforced concrete, stone and wood structures.

The given documents represent the first generation of procedures on assessment of seismic hazard and purpose of building state performance characteristics. They regulate the use of the following safety insurance levels approved for structural and non-structural buildings elements:

the further safe building operation after earthquake [Operation level];

the opportunity for immediate occupancy [Immediate Occupancy];

the level at which the repair works are allowed [Damage Control];

the level which is characterized by life safety [Life Safety];

the level of limited safety [Limited Safety];

appearance of facility structural instability (collapse) [Structural Stability];

the level which is not considered (nonconstructive assessments) [Not Considered].

For practical application it is possible to use a set of “performance characteristics” which corresponds to information on seismicity of certain regions and their correspondence to seismic zoning maps with determined levels of impacts and possible earthquakes. Taking into account this thesis having seismic knowledge on earthquakes effects in Ukraine at buildings and facilities design for practical purposes it is enough to take three levels of seismic resistance which should correspondent to structures damages which are given in Figure 1 and characterize the following:

no damages and opportunity to continue the building operation after earthquake [Immediate Occupancy] - weak earthquake (WE)

life safety and opportunity to perform the repair works after moderate earthquake [Life Safety] - design-basis earthquake (DBE)

facility stability, safety of people, valuable equipment and infrastructure which are necessary to eliminate the consequences of earthquake [Structural Stability] - maximum design earthquake (MDE).

The specific values of seismic hazard and load parameters for each country are given in National Annexes in accordance with the general provisions of EN 1998-1 (Eurocode 8, 2004).

3. Results

The results of experiments (Figure 2) and analysis of earthquakes consequences (Uzdin, et al., 1993; Chopra, 2005) showed that inelastic response spectrum depends on vibrations characteristics which are expected on the site and nonlinear materials characteristics and constructive schemes of buildings and facilities. Thus, inelastic response spectrum for determined influence should consider hysteresis characteristics which correspond to expected state of the used materials and structures.

Fig. 2:

General view for fragment of 9-storey large-panel building (a) and scheme of cracks in panels walls (b) at alternate static load testing.

The approach to update the elastic normative spectrum using the reduction coefficient R _μ is based on works of N.Newmark and W.Hall (1982), A.Сhopra (2005) and at present it is used in different seismic codes: EN 1998-1 (Eurocode 8, 2004), ATC 40 (Newmark et al., 1982), FEMA-273 (NEHRP, 1997b), FEMA-356 (Prestandard.., 2000).

According to (Chopra, 2005; Aizenberg, 1981) the dependence between structure reduction coefficient R _μ , ductility coefficient μ and period of natural vibrations Т _n is as follows:

(1)

.

where T _а , T _b и T _с are bonders of zones which correspond to the dynamic system response to accelerations, velocity and displacements at earthquake.

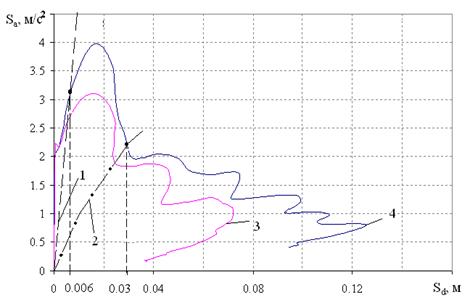
Dependences (1) were used to build up the graphs of dynamic response factors and inelastic response spectra which help to determine the seismic loading on buildings and facilities and their nonlinear displacements (Nemchinov, 2011) on the basis of spectral method given in DBN В.1.1-12:2006 (Construction in.., 2006). Figure 3 shows the dependences of spectral accelerations S _a on spectral displacements S _d which are built up taking into account the DBN В.1.1-12:2006 (Construction in.., 2006), spectral dynamic response factors graphs for soils of the first, second and third categories considering the seismic characteristics and earthquake intensity of 7 points on scale of seismic intensity in Ukraine (Aizenberg, 1981). At μ=1, 2, 4, 6 there are 1, 2, 3, 4, respectively.

Fig. 3:

Dependence “S _a -S _d ” at different μ for soils of the 1-st (а), 2-nd (b) and 3-rd (c) categories (7 points on scale (Protection against dangerous geological processes, dangerous operation influence and fire, 2011)).

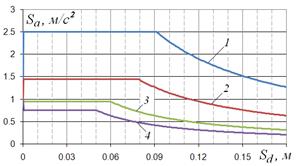
Figure 4 shows an example to determine the nonlinear displacements of three buildings of various constructive schemes where the values of natural vibrations period (the first form Т ₁ ) and ductility coefficient μ are as follows:

6-storey monolithic building (period Т ₁ = 0,37 s, μ = 1,28);

9-storey large-panel building (period Т ₁ = 0,7 s, μ = 4);

7-storey frame building (period Т ₁ = 1,0 s, μ = 1,7).

The nonlinear displacements for buildings 1, 2 and 3 are d ₁ =0,038 m, d ₂ =0,12 m and d ₃ =0,16 m, respectively. The nonlinear displacements can be determined by the Equation 2, where ω is the equivalent single-mass building model frequency (oscillator, rad/s).

(2)

,

Table 1 shows the results of calculation on maximal displacements of buildings of various constructive schemes obtained on the basis of inelastic response spectra which are given in this report and their comparison with the results of full-scale dynamic testing by powerful vibration machines (Itskov et al., 1984; Zolotkov, 2000) and records made during the past earthquakes (Peter, et al., 2000).

Table 1

Comparison of actual and calculated values of maximal buildings tops displacements

Fig. 4:

Examples to determine the nonlinear displacements d of three buildings (1, 2 and 3) with different values of period Т₁, yield limit and ductility coefficient for soils of the 2-nd category considering the seismic characteristics at earthquake intensity of 9 points on scale (Protection against dangerous geological processes, dangerous operation influence and fire ,2011).

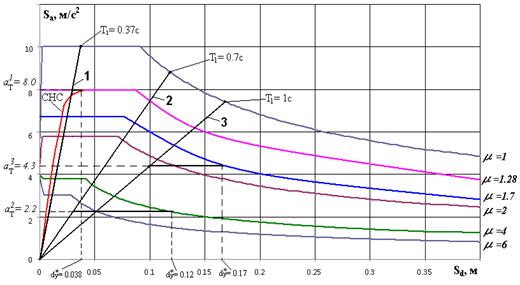
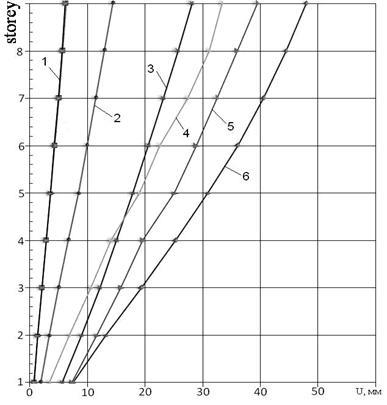
Figure 5 and Table 2 show the results of calculations on maximal displacements of buildings of various constructive schemes obtained by methodology of EN 1998-1 (Eurocode 8, 2004) and inelastic response spectra given in this report.

Figure 6 shows the relations of natural vibrations period of 9-storey building (Т _с =0,65 s) and predominated periods of thirty accelerograms (9 points) registered during the earthquakes in the USA. The calculations are performed for single-mass equivalent system (its parameters are determined on the basis of load-bearing capacity spectrum method. Figure 6 shows that expected maximal calculated vibrations of building depend on spectral composition of ground accelerations during the earthquake. At given accelerograms of construction site during calculations it is important to consider changing of building dynamic characteristics (periods and forms of natural vibrations) caused by degradation of structure stiffness during the intensive earthquakes in accordance with graphs in Figure 7.

Fig. 5:

Determination of linear (line 1) and non-linear (2) displacements of 9-storey building at earthquake intensity of 8 points (3 and 4 are accelerograms spectra).

Table 2

Comparison of calculated values of maximal reinforced concrete buildings top displacements

Fig. 6:

Dependencies of non-linear horizontal vibrations amplitudes of 9-storey large-panel building with cracks in reinforced concrete panels (period Т_с=0,65 s) on predominated periods of accelerograms (M - mathematical expectation).

Fig. 7:

Horizontal displacements of storeys during the non-linear and linear calculations of 9-storey large-panel building: 1 - Spectral (linear); 2 - Non-linear (statics); 3 - Non-linear (statics) with cracks; 4 - Results of experiment; 5 - Non-linear (dynamics);6 - Non-linear (dynamics) with cracks.

4. Discussion

Methodology to calculate the non-linear displacements of buildings

The methodology to calculate the seismic response (non-linear displacements) of buildings on the basis of load-bearing capacity spectrum (BCS) includes the following stages:

Usage of software to form design multimass three-dimensional model of the building on the basis of design or actual data in accordance with the results of structures surveys during the assessment of earthquake resistance of the existing building with damages caused by earthquakes. Diagrams of concrete and reinforcement state and cracks in load-bearing structures of superstructure and foundation are considered.

Calculation of multi-mass building model for seismic loading in linear formulation using the spectral methodology which determines the following:

masses at each i level of the model throughout the height;

frequencies (periods) by j form of vibrations;

ordinates by j form of vibrations;

inertial (seismic) loads, S _ji , for i level of the building calculation scheme by j form of vibrations.

Distribution of inertial loads, S _ji , by j form of vibrations is taken as external action to perform the non-linear static calculation of three-dimensional building model. Inertial loads S _ji , by j form of vibrations are applied step by step at each i level of the model throughout the height.

New method to determine the plane stress wall and framed reinforced concrete structures stiffness taking into account the inclined cracks. It is based on unit bands method using the compound bars theory developed by A.R. Rzhanitsyn. The actual scheme of cracks (according to operational building survey results) or scheme of “envelope” (according to earthquake consequences analysis results) (see Figure 8) is used for the calculated structure. The vertical unit band which is calculated using the scheme of compound bar with monolithic joints without cracks and with collapsible joints with cracks in the structure is cut (Figure 9) using the method of sections. The vertical unit band efforts work, W ₁ , is determined (if finite elements method is used, the single dimension is replaced by value of Δx) not considering the cracks and the vertical unit band efforts work, W ₂ , is determined considering the cracks.

Fig. 8:

Calculation of plane stress reinforced concrete structures with openings for seismic action: 1 - limits of horizontal bands; 2 - cracks; 3 - opening.

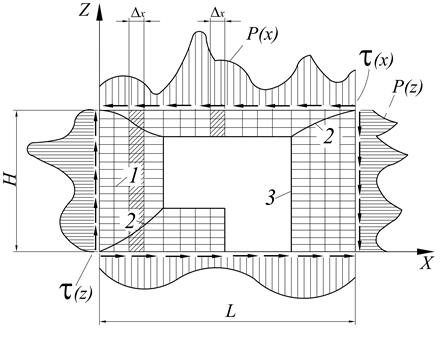
For practical calculations it is allowed to determine the thickness of finite elements which are adjacent to cracks using the difference of works of only two finite elements which are adjacent to horizontal and vertical lengths of modelled crack.

Fig. 9:

The vertical unit band which is considered using the scheme of compound bar:

1 - transverse joints; 2 - cracks.

Difference of works 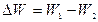is distributed for near located elements which are adjacent to cracks at the top and at the bottom. New values for thickness, b ₂ , of finite elements which are adjacent to cracks are determined by Equation 2, where - sum of works in horizontal bands which are adjacent to cracks in the limits of the vertical band; 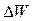- difference of works; b ₁ - initial thickness of the finite element.

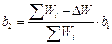
Number of vertical bands can be complete (in the limits of the structure) or partial when it is enough to use six vertical bands and intermediate values, b _k , are determined using the linear interpolation.

The methodology makes it possible to determine the stiffness of structures and storeys of buildings consisting of reinforced concrete, plane stress and framed structures with cracks using two variants. The typical scheme of cracking in these structures at alternate seismic loads in a form of mutually intersecting diagonals (scheme of “envelope”) is used. Degree of cracking is taking in accordance with seismic scale in dependence on intensity of seismic action, constructive scheme of building height or according to results of non-linear static calculation in dependence on skewed storeys.

The first variant is performed without changing the primary given order and numbers of plane finite elements, into which the plane stress structure is divided for calculation by finite elements method. The thickness of finite elements adjacent to crack-diagonals which is determined by equation of works in unit bands using the compound bar model and equivalent plane stress structure model is reduced. The reduced thickness of design structure model finite elements adjacent to virtual crack causes the actual cracking along the diagonals.

The algorithm of calculation requires the iterative process regulated by determined precise finite elements thickness and building dynamic characteristics (frequencies and forms of natural vibrations).

The second variant to determine the plane stress and framed building structures stiffness is based on special approach to model the cracks which are located on diagonal of wall panels without openings and in places of stress concentration (see Figure 8). The reinforced bars of plane stress structures are modeled by additional FE and opening and closing of cracks is considered using the computing based on finite elements method. Stiffness of framed structures on sites with inclined cracks including the intersecting cracks (typical for joints and sites observed near supports at seismic actions) is determined using the special design model of plane stress structures (Figure 10).

1

Limits of horizontal bands; 2 - cracks; 3 - absolutely stiff end insert.

The potential energy of internal forces for one of the selected zones is determined by Equation 3. Stiffness of the zones is replaced by equivalent stiffness in Equation 4. Iterative process is finished when expected precision of calculation is obtained B ₁ (λ).

(3)

.

(4)

.

On sites with normal cracks the stiffness of framed reinforced concrete structures is determined by the value of bending moment and radius of curvature, ρ, using the normative methodology for the considered i zone (site is divided into 4 - 6 zones), as shown in Equation 5.

(5)

The nonlinear static calculation (using software system which makes it possible to consider physical nonlinearity of materials) determines the values of displacements, u _in for each i level for each n step of loading. Using these values the graphs of dependencies “shear force S_i - displacement u _i ” are built for each i level (storey) of design model.

Using the above given dependencies the spectrum of building bearing capacity in coordinates “spectral acceleration S _aj - spectral displacement S _dj ” using the j form of vibrations. To convert the load-bearing capacity spectrum graph to dependency “load S _base - displacement S _d ” the modal (equivalent) mass is multiplied by value of spectral acceleration S _aj .

5. Conclusions and recommendations

The inelastic response spectra in coordinates “β - Т” based on spectral dynamic coefficients graphs given in norms of Ukraine DBN В.1.1-12:2006 and in coordinates “Sa - Sd” developed to perform the nonlinear calculations of buildings structures at design and assessment of used buildings earthquake resistance using the nonlinear static methods of calculation are obtained.

Comparison of the values for maximal displacements of buildings of various constructive schemes obtained at realization of full-scale dynamic testing and measuring of buildings vibrations during the earthquakes with the results of calculation using the developed methodology on the basis of proposed inelastic dynamic response spectra showed a good correspondence. The maximal error is 11%.

The values of maximal top displacements of the buildings of various constructive schemes obtained by calculation using the procedure given in Attachment B of EN 1998-1 and the proposed methodology on the basis of inelastic dynamic response spectra are different by 10%.

The developed methodology is recommended to be used at assessment of earthquake resistance of buildings designed and operated in seismic regions after the main shock and for further aftershocks (considering the existing cracks in load-bearing structures and physical nonlinearity of concrete and reinforcement) and at design of responsible facilities and buildings using new constructive solutions which are not checked during the strong earthquakes.